Carsten Frederiksen / Matteo Di Manno (Sapienza University of Rome) in cooperation with Annalisa Fregolent and students of the University of L'Aquila, Jacopo Brunetti, and Walter D'Ambrogio.

Thursday, November 21, 2024 · 0 min read

Identification of the Physical Parameters of Bolted Joints

For mechanical structures composed of several components connected by joints, the study of the joint is of fundamental importance. Indeed, identifying the joint parameters allows for studying the effect of the joints on the dynamic behaviour of the assembled system. One identification technique involves so-called decoupling. This technique requires measuring the frequency response functions of the system assembly and its components.

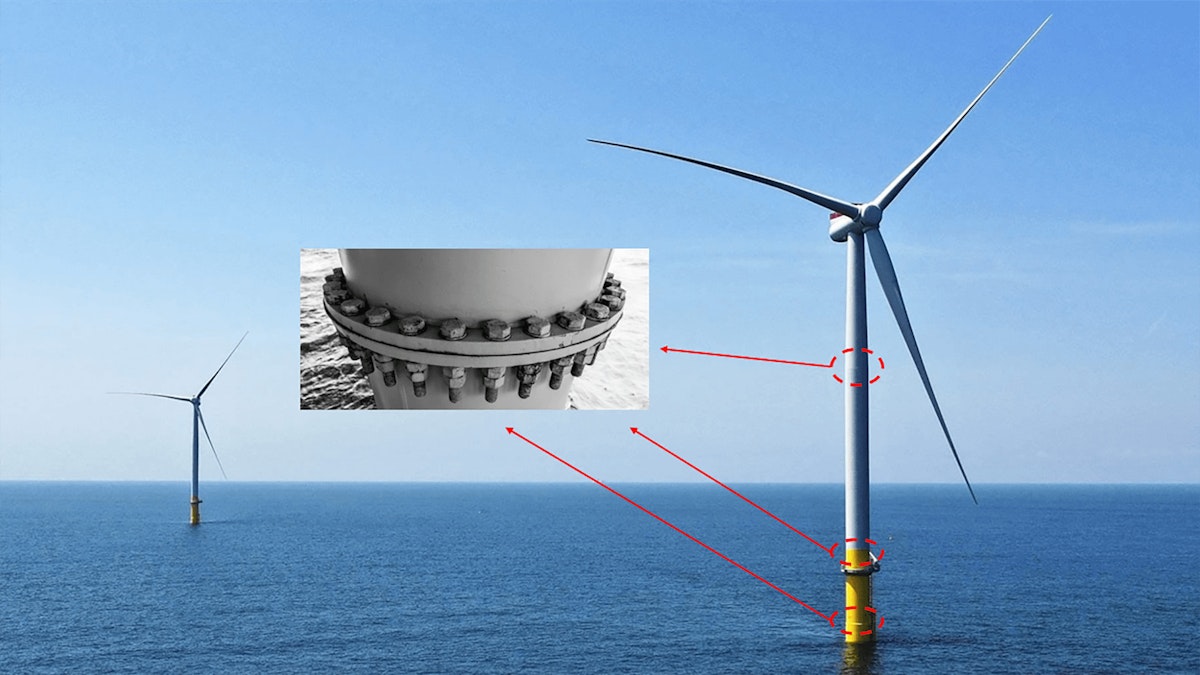
A group of students at two Italian universities, the Sapienza University of Rome and the University of L'Aquila, set out to experiment with the decoupling technique. They joined research teams in mechanical vibration and vibroacoustics. 

Most mechanical structures are composed of several components connected by joints, such as bolted or nailed joints, etc. Although the joints are located in only a small part of the structure, they can significantly influence the dynamic behaviour of the assembled system. 

A striking example is that of bolted joints, which can modify the overall stiffness of the structure in the area interfacing the components. As a consequence, a variation of the natural frequencies of the assembled system occurs depending on the operating conditions. This, for example, is observed in wind turbine towers.

Studying the effect of mechanical joints on the dynamic behaviour of the assembled system is therefore of fundamental importance. Starting from the analysis of a simplified system such as the one in Figure 1.

Figure 1. Commonly used benchmark for the study of bolted joints.

In structural dynamics, such studies can be done by modelling the joint with mass, stiffness, and damping parameters that connect subsystems and components. Since these parameters are not known a priori, they must be identified through a procedure known as joint identification.

Joint identification by decoupling

One of the most used methods to identify joints is that of dynamic substructuring, in particular, the procedure called decoupling. In substructuring, an assembled structure is decomposed into several connected component subsystems. 

Consider, for example, an assembled structure in which a bolted joint connects two components (see Figure 2). This jointed structure can be decomposed into three subsystems, the two-component subsystems A and B, and a third subsystem, which represents the bolted joint J. The decoupling allows identifying the dynamic behaviour of the jointed subsystem J, removing from the dynamics of the assembled system AJB, the dynamics of subsystems A and B measured individually.

Figure 2. Joint identification procedure by decoupling.

To identify the joint through decoupling, you have to measure the Frequency Response Functions (FRF) of the assembled structure and the individual component subsystems. FRF is defined as the ratio between response and excitation as a function of the excitation frequency.

Experimental application

In our experiment, we used decoupling to identify the bolted joint of a benchmark known in the literature as a Brake Reuss Beam, two steel beams connected at the mid-span by three bolts.

Figure 3. Brake Reuss Beam benchmark.

The frequency response functions of the assembled system and single beams were acquired using the DewesoftX software's Modal Testing plug-in. 

This plug-in contains the main tools for acquiring the FRFs, with the possibility of displaying the FRFs and the coherence in real-time to evaluate the resulting quality during the measurement. Furthermore, the plug-in can extract information on the tested structure, such as natural frequencies, damping, and modal deformations.

Figure 4. Acquisition of the FRF of the assembled system.

Through 42 excitation points and 42 measurement points, we determined the frequency response functions of the assembled system. We used a SIMO (Single Input-Multi Output) test procedure in which the structure was excited using a modal hammer (PCB 086C03), and we measured the acceleration at seven different points with uniaxial accelerometers (PCB 352A24). 

The force and acceleration were recorded using a Dewesoft SIRIUSi acquisition system with eight analog channels, based on DualCoreADC® technology with a double 24-bit analog-to-digital converter. 

An anti-aliasing filter on each analog channel allowed us to reach a dynamic range of 160 dB in time and frequency with a sampling frequency of 200 kHz. In this case, we used a sampling frequency of 5000 Hz to measure the FRF in the 0-1400 Hz range. 

We applied the roving hammer technique available in the Modal Testing plug-in to measure all frequency response functions. Once the positions of the seven accelerometers were fixed, we excited the structure in all 42 excitation points. This procedure was repeated by moving the accelerometer positions without interrupting the measurement.

The Modal Testing plug-in also allowed us to analyze the measurements made in the post-processing phase. Figure 5 shows some of the FRFs measured for the assembled system.

Figure 5. Measured frequency response functions for the assembled system with their coherence.

Once we had also measured the FRF of the single component beams, it was possible to identify the mass and stiffness parameters of the joint by applying the decoupling procedure. For example, we found an overall stiffness value of 9.4e9 N/m and one of 3.1e10 N/m for the contact stiffness at the central bolt.

Conclusions

In the decoupling application, the DewesoftX modal testing plug-in enabled us to acquire a large number of frequency response functions in a short time. We were able to do this thanks to the simplicity of the software interface and the possibilities of using the roving hammer technique and the display of FRF in real-time. The consistency of the software and the plug-in also allowed us to verify the validity of the measurements immediately.